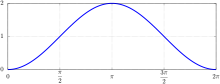
正矢
 | |
| 性質 | |
| 奇偶性 | 偶 |
| 定義域 | (-∞,∞) |
| 到達域 | [0,2] |
| 周期 | (360°) |
| 特定值 | |
| 當x=0 | 0 |
| 當x=+∞ | N/A |
| 當x=-∞ | N/A |
| 最大值 | (, 2) (360°k+180°, 2) |
| 最小值 | (, 0) (360°k, 0) |
| 其他性質 | |
| 渐近线 | N/A |
| 根 | () |
| 臨界點 | () |
| 拐點 | () |
| 不動點 | 0 |
| k是一個整數。 | |

正矢(英文:versine、versed sine)是一種三角函數,出現於早期的三角函數表(如梵语的阿耶巴塔三角表[1]第一節),其值為1和餘弦函數的差。它的定义域是整个实数集,值域在0到2之間。它是周期函数,其最小正周期为(360°)。在自变量为(,其中为整数)时,该函数有极大值2;在自变量为(或)时,该函数有极小值0。正矢函数是偶函数,其图像关于y轴对称。
概述
[编辑]正矢函數(versine[2][3][4][5][6]或versed sine[7][8][9][10][11]) 是一個三角函數,常計為versin(θ)、 sinver(θ)、[12][13] vers(θ)、 ver(θ)[14]或 siv(θ)。[15][16] 在拉丁語中,其被稱為sinus versus(翻轉的正弦)、 versinus、 versus或 sagitta(箭頭)。[17]
其等價定義為:
相關函數
[编辑]- 餘的正矢(英文:versed cosine、vercosine)[18],寫為vercosin(θ)、 vercos(θ)或 vcs(θ)。
- 餘矢(英文:coversed sine、coversine)[19],寫為,有時亦縮寫為。
- 餘的餘矢(英文:coversed cosine[20]或 covercosine),寫為covercosin(θ)、 covercos(θ)或 cvc(θ)。
與上述四個函數類似,還存在四個“半值”函數:
- 半正矢(英文:haversed sine,[21] haversine或 semiversus[22][23]),寫為haversin(θ)、 semiversin(θ)、 semiversinus(θ)、 havers(θ)、 hav(θ)、[24][25] hvs(θ)、[註 1] sem(θ)或 hv(θ)[26],因半正矢公式出名,且曾用於導航術。
- 餘的半正矢(英文:haversed cosine[27]或 havercosine),寫為havercosin(θ), havercos(θ), hac(θ)或 hvc(θ),其可運用在訊號處理和機率統計上,參見餘的半正矢應用一節。
-
- 半餘矢(英文:hacoversed sine、hacoversine[28]或 cohaversine),寫為hacoversin(θ)、 semicoversin(θ)、 hacovers(θ)、 hacov(θ)[29]或 hcv(θ)。
- 餘的半餘矢(英文:hacoversed cosine[30]、 hacovercosine或 cohavercosine),寫為hacovercosin(θ)、 hacovercos(θ)或 hcc(θ)。
歷史與應用
[编辑]正矢和餘矢
[编辑]

普通的正弦函數在歷史上有時被稱為sinus rectus (「straight sine」,直譯「直正弦」),以與「versed」的正弦函數,即正矢函數(sinus versus)進行對比[32]。如果在原始上下文中檢視函數的定義(單位圓),這些術語的含義就會很明顯:
- 對於單位圓的垂直弦AB,角θ(代表上下角Δ[註 2]的一半)的正弦是距離AC(垂直弦的一半)。另一方面,θ的正矢是從垂直弦的中心到圓弧中心的距離CD。因此,cos(θ)(等於線OC的長度)和versin(θ)(等於線CD的長度)總和就是半徑OD(長度為單位長)。以這種方式說明,正弦是垂直的,而正矢是水平的(正矢又稱為sinus versus,其中versus字面意思是「相反、不合適」); 兩者都是從點C到圓周的距離,僅差在一個是垂直(正弦),一個是水平(正矢)。
這張圖也說明了為什麼正矢有時被稱為sagitta(拉丁語中箭頭的意思)[17][31],源自阿拉伯語用法sahem[33],具有相同的含義。 它本身來自印度單字「sara」(箭頭),通常用來指「utkrama-jya」。 如果把上下角[註 2]Δ=2θ的弧ADB看成是「弓」,弦AB看成是「弦」,那麼正矢對應的線段CD顯然就是「箭矢」。
為了進一步與將正弦解釋為「垂直」並且將正矢解釋為「水平」保持一致,「矢」一字(sagitta)在此也可以視為橫座標(圖中的水平軸)的過時同義詞。[31]
1821年,奧古斯丁-路易·柯西使用術語「sinus versus」(siv)表達正矢,以及使用「cosinus versus」(cosiv)表達餘矢[15][16](不過部分文獻混淆了「餘的正矢」與「餘矢」,柯西在1821年的文獻中並未討論到「餘的正矢」,因此它們可能起源於較晚的時間)[註 3]。

從歷史上看,正矢函數被認為是十分重要的三角函數之一。[11][32][33]
當角θ十分接近於0的時候,正矢versin(θ)的值會是兩個幾乎相等的值的差,因此僅使用餘弦函數的三角函數表來計算的話,該表需要非常高的精度才能在避免灾难性抵消對於計算正矢函數時所造成的數值誤差,因此製作專用於正矢函數的三角函數表是有必要的。[11]即使是使用计算器或计算机,由於舍入誤差,對於較小的θ,也會建議使用sin2公式來計算正矢值。
正矢的另一個歷史優勢是它的函數值總是非負的,因此除了單一特殊的角度(如θ = 0, 2π, …及其同界角)為零之外,正矢函數的對數在任何地方都有定義,因此,乘法有涉及正矢函數的計算可以使用對數表來計算。
事實上,現存最早的正弦(半弦長)值表(與托勒密和其他希臘作家列出的弦函數表相對)是印度的Surya Siddhantha計算得出的,其可追溯到公元前3世紀,這個表是一個紀錄了從0到90°之每3.75°的正弦和正矢數值的表。[32]
正矢是應用半角公式sin2(θ/2) = 1/2versin(θ)的中間步驟,該公式由托勒密導出,用於建立此類數學用表。
半正矢
[编辑]半正矢函數出現於半正矢公式中,其可以据两点的经度和纬度来确定大圆上两点之间距离,且在導航術中被廣泛地使用,因此十九和二十世纪初的导航和三角测量书中包含了半正矢值表和对数表。[34][35][36]1835年,詹姆斯·英曼[13][37][38]在其著作《航海与航海天文学:供英国海员使用》(Navigation and Nautical Astronomy: For the Use of British Seamen)第三版中创造了“半正矢”一词[39]以简化地球表面两点之间的距离计算,應用於球面三角学關於导航的部分。[2]
現代用途
[编辑]雖然正矢、餘矢和半正矢及其反函數的使用可以追溯到幾個世紀前,但其他五個餘函數的名稱(餘的正矢、餘的餘矢、半餘矢、餘的半正矢、餘的半餘矢)似乎較晚才出現。
即便如此,這些函數到了近代都還是存在相關應用,例如正矢的相關函數半正矢有應用在一些少見領域的計算方法上,以及餘的半正矢的相關函數則運用於訊號處理、控制理論、機率論和統計學中。
其中正矢的半值函數——半正矢函數在近幾十年來發現了新的應用。如1995年來布魯斯·D·斯塔克(Bruce D. Stark)利用高斯對數之清晰的月角距計算方法[40][41],以及2014年提出用於視線縮減之更緊湊的方法[26]。
餘的半正矢
[编辑]餘的半正矢(havercosine)是餘的正矢(vercosine)之半值函數,其定義為:
一個週期(0 < θ < 2π)的正弦或更常見的餘的正矢(vercosine)之半值函數(havercosine)波形也常用於訊號處理和控制理論中,作為脈衝或窗函數的形狀(包括漢恩窗、漢恩–泊松窗和圖基窗),因為它平滑地(在值和斜率上連續)從0遞增到1(對於半正矢)再對稱地遞減回0。[註 1]在這些應用中,它被稱為漢恩函數或升餘弦濾波器。 同樣,餘的正矢(vercosine)之半值函數(havercosine)也用於機率論和統計學的升餘弦分佈[42]。
結語
[编辑]綜觀下來,正矢函數被認為是十分重要的三角函數之一[11][32][33],圍繞其的餘函數——餘矢函數亦是如此。主要是為了在计算器與计算机發明之前高度仰賴三角函數表來計算的時代,避免出現灾难性抵消對於計算正矢函數以及餘矢函數時所造成的數值誤差[11]。後來發現其對應的半值函數——半正矢能運用在導航術上因此被廣泛使用。相應的每個函數都有其餘函數,在這些函數發展的晚期,這些其餘函數——如餘的正矢、餘的餘矢、半餘矢、餘的半正矢、餘的半餘矢才逐漸被拿來討論。但到了计算器與计算机發明之後,這些函數的需求逐漸式微,因此變得較少使用,但在特定領域仍有發揮空間,如餘的半正矢能應用在訊號處理與機率統計上。
數學性質
[编辑]定義
[编辑]正矢、餘矢、餘的正矢、餘的餘矢、半正矢、半餘矢、餘的半正矢、餘的半餘矢定義如下:
| 正矢 | [3] | 
|
|---|---|---|
| 餘矢 | [3] | 
|
| 餘的正矢 | [18] | 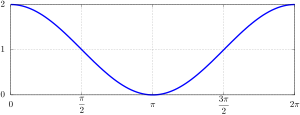
|
| 餘的餘矢 | [20] | 
|
| 半正矢 | [3] | 
|
| 半餘矢 | [28] | 
|
| 餘的半正矢 | [27] | 
|
| 餘的半餘矢 | [30] | 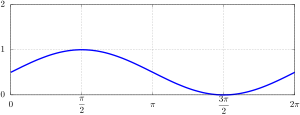
|
圓週旋轉
[编辑]這些函數具備圓週旋轉性值。例如正矢和餘矢即角度差90度、正矢和餘的正矢角度差180度、正矢和餘的餘矢角度差270度,以此類推。半值函數亦然。
微分與積分
[编辑]| [43] | [3][43] |
| [19] | [19] |
| [21] | [21] |
反函數
[编辑]這些函數也存在對應的反函數:
- 反正矢(arcversine)[29]:正矢的反函數,又可以記作arcversin、 arcvers[7][29]、 avers[44][45]或aver。
- 反餘的正矢(arcvercosine):餘的正矢的反函數,又可以記作arcvercosin、 arcvercos、 avercos或avcs。
- 反餘矢(arccoversine)[29]:餘矢的反函數,又可以記作arccoversin、 arccovers[7][29]、 acovers[44][45]或acvs。
- 反餘的餘矢(arccovercosine):餘的餘矢的反函數,又可以記作arccovercosin、 arccovercos、 acovercos或acvc。
- 反半正矢(archaversine):半正矢的反函數,又可以記作archaversin、 archav[29] 、 haversin−1[46]、 invhav[29][47][48][49]、 ahav[29][44][45]、 ahvs、 ahv或hav−1[50][51]。
- 反餘的半正矢(archavercosine):餘的半正矢的反函數,又可以記作archavercosin、 archavercos或ahvc。
- 反半餘矢(archacoversine):半餘矢的反函數,又可以記作archacoversin或ahcv。
- 反餘的半餘矢(archacovercosine):餘的半餘矢的反函數,又可以記作archacovercosin、 archacovercos或ahcc。
| [29][44][45] |
| [29][44][45] |
| [29][44][45][46][47][48][50][51] |
其他性質
[编辑]
近似值
[编辑]

當v的正矢函數值versine v與半徑r相比較小時,可以透過以下公式從半弦長度L(上圖所示的AC長)近似得出正矢值:[52]
或者,如果正矢函數值很小,且已知正矢函數值、半徑和半弦長,則可以透過以下公式來估計計弧長s(上圖所示的AD):
這個公式為中國數學家沈括所知,兩個世紀後,郭守敬提出了一個更準確的公式,也涉及弦弧間最大的距離。[53]
工程中使用的更準確的近似是[54]:
任意曲線和弦
[编辑]術語「正矢」(versine)有時也用來描述任意平面曲線中弦與曲線間最大的距離,上面的圓是其中的一個特例。 給定曲線中兩點之間的弦,從弦到曲線(通常在弦中點)的垂直距離v稱為正矢測量(versine measurement)或軌道曲線正矢測量[55]。對於直線,任何弦的正矢為零,因此該測量表徵了曲線的直線度。在極限情況下,當弦長L趨近於零時,瞬時曲率的比率為8v/L2。 這種用法在鐵路運輸中尤其常見,它描述了鐵軌直線度的測量[56],並且它是鐵路測量的哈拉德方法之基礎。
其他相關函數
[编辑]
正矢的值域範圍在0到2之間,類似的函數還有弦函數(crd),值域範圍也在0到2之間,但函數圖形略有差異。正矢函數的圖形與正弦函數的形狀相同,但x軸與y軸都平移了一段距離。正矢函數與其他「正」的三角函數(正弦、正切、正割)同樣是從零開始遞增的函數。
參見
[编辑]註釋
[编辑]- ^ 1.0 1.1 在訊號分析中,hvs有時用於半正矢函數(haversine function),也有時代表单位阶跃函数。
- ^ 2.0 2.1 2.2 對於圓心在原點之單位圓上的角θ,如果角θ的始邊是x軸,那麼角對應的正弦線段可以穿過x軸向下延長直到交於圓上另一點,形成一個以x軸對稱的另外一個角。若原始角θ稱為上角,下方對稱於x軸鏡像的角稱為下角,那麼這兩個角的正弦線段接組成成一個完整的以x軸對稱的弓形的弦,這上角與下角之和則正好為2θ,因此為了方便描述與計算,就將此「上下角」以Δ表示,則有Δ=2θ。
- ^ 一些英文文獻將「餘的正矢」(versed cosine)與「餘矢」(coversed sine)混淆了。歷史上(如柯西在1821年發表的著作[15])曾將sinus versus(versine,正矢)定義為siv(θ) = 1−cos(θ),且cosinus versus(coversed sine,餘矢)定義為cosiv(θ) = 1−sin(θ),而vercosine(餘的正矢)定義為vcs(θ) = 1+cos(θ)。然而,在2009年柯西著作的英文翻譯中,布蘭得利(Bradley)和桑迪弗(Sandifer)[16]將cosinus versus(cosiv,即餘矢)與餘的正矢(versed cosine,又稱vercosine)聯繫起來,而不是與其對應定義的函數coversed sine(餘矢),因此兩者被混淆了。類似的現象也發生在1968年/2000年的著作上,柯恩[10]將covers(θ)(餘矢函數)與餘的正矢(versed cosine)相聯繫,而不是與其對應定義的函數coversed sine(餘矢)。
參考文獻
[编辑]- ^ The Āryabhaṭīya by Āryabhaṭa
- ^ 2.0 2.1 Inman, James. Navigation and Nautical Astronomy: For the Use of British Seamen 3. London, UK: W. Woodward, C. & J. Rivington. 1835 [1821] [2015-11-09]. (原始内容存档于2022-05-27). (Fourth edition: [1] (页面存档备份,存于互联网档案馆).)
- ^ 3.0 3.1 3.2 3.3 3.4 Zucker, Ruth. Chapter 4.3.147: Elementary Transcendental Functions - Circular functions. Abramowitz, Milton; Stegun, Irene Ann (编). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. Applied Mathematics Series 55 Ninth reprint with additional corrections of tenth original printing with corrections (December 1972); first. Washington D.C.; New York: United States Department of Commerce, National Bureau of Standards; Dover Publications. 1983: 78. ISBN 978-0-486-61272-0. LCCN 64-60036. MR 0167642. .
- ^ Tapson, Frank. Background Notes on Measures: Angles. 1.4. Cleave Books. 2004 [2015-11-12]. (原始内容存档于2007-02-09).
- ^ Oldham, Keith B.; Myland, Jan C.; Spanier, Jerome. 32.13. The Cosine cos(x) and Sine sin(x) functions - Cognate functions. An Atlas of Functions: with Equator, the Atlas Function Calculator
 2. Springer Science+Business Media, LLC. 2009: 322 [1987]. ISBN 978-0-387-48806-6. LCCN 2008937525. doi:10.1007/978-0-387-48807-3.
2. Springer Science+Business Media, LLC. 2009: 322 [1987]. ISBN 978-0-387-48806-6. LCCN 2008937525. doi:10.1007/978-0-387-48807-3.
- ^ Beebe, Nelson H. F. Chapter 11.1. Sine and cosine properties. The Mathematical-Function Computation Handbook - Programming Using the MathCW Portable Software Library 1. Salt Lake City, UT, USA: Springer International Publishing AG. 2017-08-22: 301. ISBN 978-3-319-64109-6. LCCN 2017947446. S2CID 30244721. doi:10.1007/978-3-319-64110-2.
- ^ 7.0 7.1 7.2 7.3 7.4 Hall, Arthur Graham; Frink, Fred Goodrich. Review Exercises [100] Secondary Trigonometric Functions. 写于Ann Arbor, Michigan, USA. Trigonometry. Part I: Plane Trigonometry. New York, USA: Henry Holt and Company / Norwood Press / J. S. Cushing Co. - Berwick & Smith Co., Norwood, Massachusetts, USA. January 1909: 125–127 [2017-08-12].
- ^ Boyer, Carl Benjamin. 5: Commentary on the Paper of E. J. Dijksterhuis (The Origins of Classical Mechanics from Aristotle to Newton). Clagett, Marshall (编). Critical Problems in the History of Science 3. Madison, Milwaukee, and London: University of Wisconsin Press, Ltd. 1969: 185–190 [1959] [2015-11-16]. ISBN 0-299-01874-1. LCCN 59-5304. 9780299018740.
- ^ Swanson, Todd; Andersen, Janet; Keeley, Robert. 5 (Trigonometric Functions) (PDF). Precalculus: A Study of Functions and Their Applications. Harcourt Brace & Company. 1999: 344 [2015-11-12]. (原始内容存档 (PDF)于2003-06-17).
- ^ 10.0 10.1 Korn, Grandino Arthur; Korn, Theresa M. Appendix B: B9. Plane and Spherical Trigonometry: Formulas Expressed in Terms of the Haversine Function. Mathematical handbook for scientists and engineers: Definitions, theorems, and formulars for reference and review
 3. Mineola, New York, USA: Dover Publications, Inc. 2000: 892–893 [1961]. ISBN 978-0-486-41147-7. (See errata.)
3. Mineola, New York, USA: Dover Publications, Inc. 2000: 892–893 [1961]. ISBN 978-0-486-41147-7. (See errata.)
- ^ 11.0 11.1 11.2 11.3 11.4 Calvert, James B. Trigonometry. 2007-09-14 [2004-01-10] [2015-11-08]. (原始内容存档于2007-10-02).
- ^ Edler von Braunmühl, Anton. Vorlesungen über Geschichte der Trigonometrie - Von der Erfindung der Logarithmen bis auf die Gegenwart [Lectures on history of trigonometry - from the invention of logarithms up to the present] 2. Leipzig, Germany: B. G. Teubner. 1903: 231 [2015-12-09]. (原始内容存档于2022-05-26) (德语).
- ^ 13.0 13.1 13.2 13.3 Cajori, Florian. A History of Mathematical Notations 2 2 (3rd corrected printing of 1929 issue). Chicago, USA: Open court publishing company. 1952: 172 [March 1929] [2015-11-11]. ISBN 978-1-60206-714-1. 1602067147.
The haversine first appears in the tables of logarithmic versines of José de Mendoza y Rios (Madrid, 1801, also 1805, 1809), and later in a treatise on navigation of James Inman (1821). See J. D. White in Nautical Magazine (February and July 1926).
(NB. ISBN and link for reprint of 2nd edition by Cosimo, Inc., New York, USA, 2013.) - ^ Shaneyfelt, Ted V. 德博士的 Notes About Circles, ज्य, & कोज्य: What in the world is a hacovercosine?. Hilo, Hawaii: University of Hawaii. [2015-11-08]. (原始内容存档于2015-09-19).
- ^ 15.0 15.1 15.2 Cauchy, Augustin-Louis. Analyse Algébrique. Cours d'Analyse de l'Ecole royale polytechnique 1. L'Imprimerie Royale, Debure frères, Libraires du Roi et de la Bibliothèque du Roi. 1821 (法语).access-date=2015-11-07--> (reissued by Cambridge University Press, 2009; ISBN 978-1-108-00208-0)
- ^ 16.0 16.1 16.2 Bradley, Robert E.; Sandifer, Charles Edward. Buchwald, J. Z. , 编. Cauchy's Cours d'analyse: An Annotated Translation. Sources and Studies in the History of Mathematics and Physical Sciences. Cauchy, Augustin-Louis (Springer Science+Business Media, LLC). 2010-01-14: 10, 285 [2009] [2015-11-09]. ISBN 978-1-4419-0548-2. LCCN 2009932254. doi:10.1007/978-1-4419-0549-9. 1441905499, 978-1-4419-0549-9. (原始内容存档于2016-06-24). (See errata.)
- ^ 17.0 17.1 17.2 van Brummelen, Glen Robert. Heavenly Mathematics: The Forgotten Art of Spherical Trigonometry. Princeton University Press. 2013 [2015-11-10]. ISBN 9780691148922. 0691148929.
- ^ 18.0 18.1 Weisstein, Eric W. (编). Vercosine. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. [2015-11-06]. (原始内容存档于2014-03-24) (英语).
- ^ 19.0 19.1 19.2 19.3 Weisstein, Eric W. (编). Coversine. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. [2015-11-06]. (原始内容存档于2005-11-27) (英语).
- ^ 20.0 20.1 Weisstein, Eric W. (编). Covercosine. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. [2015-11-06]. (原始内容存档于2014-03-28) (英语).
- ^ 21.0 21.1 21.2 21.3 Weisstein, Eric W. (编). Haversine. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. [2015-11-06]. (原始内容存档于2005-03-10) (英语).
- ^ Fulst, Otto. 17, 18. Lütjen, Johannes; Stein, Walter; Zwiebler, Gerhard (编). Nautische Tafeln 24. Bremen, Germany: Arthur Geist Verlag. 1972 (德语).
- ^ Sauer, Frank. Semiversus-Verfahren: Logarithmische Berechnung der Höhe. Hotheim am Taunus, Germany: Astrosail. 2015 [2004] [2015-11-12]. (原始内容存档于2013-09-17) (德语).
- ^ Rider, Paul Reece; Davis, Alfred. Plane Trigonometry. New York, USA: D. Van Nostrand Company. 1923: 42 [2015-12-08]. (原始内容存档于2022-05-28).
- ^ Haversine. Wolfram Language & System: Documentation Center. 7.0. 2008 [2015-11-06]. (原始内容存档于2014-09-01).
- ^ 26.0 26.1 Rudzinski, Greg. Ix, Hanno. Ultra compact sight reduction. Ocean Navigator (Portland, ME, USA: Navigator Publishing LLC). July 2015, (227): 42–43 [2015-11-07]. ISSN 0886-0149.
- ^ 27.0 27.1 27.2 Weisstein, Eric W. (编). Havercosine. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. [2015-11-06]. (原始内容存档于2014-03-29) (英语).
- ^ 28.0 28.1 Weisstein, Eric W. (编). Hacoversine. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. [2015-11-06]. (原始内容存档于2014-03-29) (英语).
- ^ 29.00 29.01 29.02 29.03 29.04 29.05 29.06 29.07 29.08 29.09 29.10 van Vlijmen, Oscar. Goniology. Eenheden, constanten en conversies. 2005-12-28 [2003] [2015-11-28]. (原始内容存档于2009-10-28) (英语).
- ^ 30.0 30.1 Weisstein, Eric W. (编). Hacovercosine. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. [2015-11-06]. (原始内容存档于2014-03-29) (英语).
- ^ 31.0 31.1 31.2 sagitta. 牛津英語詞典 (第三版). 牛津大學出版社. 2005-09 (英语).
- ^ 32.0 32.1 32.2 32.3 Boyer, Carl Benjamin; Merzbach, Uta C. A History of Mathematics 2. New York, USA: John Wiley & Sons. 1991-03-06 [1968] [2019-08-10]. ISBN 978-0471543978. 0471543977.
- ^ 33.0 33.1 33.2 Miller, Jeff. Earliest Known Uses of Some of the Words of Mathematics (V). New Port Richey, Florida, USA. 2007-09-10 [2015-11-10]. (原始内容存档于2015-09-05).
- ^ H. B. Goodwin, The haversine in nautical astronomy, Naval Institute Proceedings, vol. 36, no. 3 (1910), pp. 735–746: Evidently if a Table of Haversines is employed we shall be saved in the first instance the trouble of dividing the sum of the logarithms by two, and in the second place of multiplying the angle taken from the tables by the same number. This is the special advantage of the form of table first introduced by Professor Inman, of the Portsmouth Royal Navy College, nearly a century ago.
- ^ W. W. Sheppard and C. C. Soule, Practical navigation (World Technical Institute: Jersey City, 1922).
- ^ E. R. Hedrick, Logarithmic and Trigonometric Tables (Macmillan, New York, 1913).
- ^ White, J. D. (unknown title). Nautical Magazine. February 1926. (NB. According to Cajori, 1929[13], this journal has a discussion on the origin of haversines.)
- ^ White, J. D. (unknown title). Nautical Magazine. July 1926. (NB. According to Cajori, 1929[13], this journal has a discussion on the origin of haversines.)
- ^ haversine. 牛津英語詞典 (第三版). 牛津大學出版社. 2005-09 (英语).
- ^ Stark, Bruce D. Stark Tables for Clearing the Lunar Distance and Finding Universal Time by Sextant Observation Including a Convenient Way to Sharpen Celestial Navigation Skills While On Land 2. Starpath Publications. 1997 [1995] [2015-12-02]. ISBN 978-0914025214. 091402521X. (原始内容存档于2023-02-26). (NB. Contains a table of Gaussian logarithms lg(1+10−x).)
- ^ Kalivoda, Jan. Bruce Stark - Tables for Clearing the Lunar Distance and Finding G.M.T. by Sextant Observation (1995, 1997) (Review). Prague, Czech Republic. 2003-07-30 [2015-12-02]. (原始内容存档于2004-01-12).[2] (页面存档备份,存于互联网档案馆)[3]
- ^ Horst Rinne. Location-Scale Distributions – Linear Estimation and Probability Plotting Using MATLAB (PDF): 116. 2010 [2012-11-16]. (原始内容存档 (PDF)于2023-03-31).
- ^ 43.0 43.1 43.2 Weisstein, Eric Wolfgang. Versine. MathWorld. Wolfram Research, Inc. [2015-11-05]. (原始内容存档于2010-03-31).
- ^ 44.0 44.1 44.2 44.3 44.4 44.5 Simpson, David G. AUXTRIG (Fortran 90 source code). Greenbelt, Maryland, USA: NASA Goddard Space Flight Center. 2001-11-08 [2015-10-26]. (原始内容存档于2008-06-16).
- ^ 45.0 45.1 45.2 45.3 45.4 45.5 van den Doel, Kees. jass.utils Class Fmath. JASS - Java Audio Synthesis System. 1.25. 2010-01-25 [2015-10-26]. (原始内容存档于2007-09-02).
- ^ 46.0 46.1 mf344. Lost but lovely: The haversine. Plus magazine (maths.org). 2014-07-04 [2015-11-05]. (原始内容存档于2014-07-18).
- ^ 47.0 47.1 Skvarc, Jure. identify.py: An asteroid_server client which identifies measurements in MPC format. Fitsblink (Python source code). 1999-03-01 [2015-11-28]. (原始内容存档于2008-11-20).
- ^ 48.0 48.1 Skvarc, Jure. astrotrig.py: Astronomical trigonometry related functions (Python source code). Ljubljana, Slovenia: Telescope Vega, University of Ljubljana. 2014-10-27 [2015-11-28]. (原始内容存档于2015-11-28).
- ^ Ballew, Pat. Versine. Math Words, page 4. Versine. 2007-02-08 [2003] [2015-11-28]. (原始内容存档于2007-02-08).
- ^ 50.0 50.1 Weisstein, Eric Wolfgang. Inverse Haversine. MathWorld. Wolfram Research, Inc. [2015-10-05]. (原始内容存档于2008-06-08).
- ^ 51.0 51.1 InverseHaversine. Wolfram Language & System: Documentation Center. 7.0. 2008 [2015-11-05]. (原始内容存档于2023-11-08).
- ^ Woodward, Ernest. Geometry - Plane, Solid & Analytic Problem Solver. Problem Solvers Solution Guides. Research & Education Association (REA). December 1978: 359. ISBN 978-0-87891-510-1.
- ^ Needham, Noel Joseph Terence Montgomery. Science and Civilisation in China: Mathematics and the Sciences of the Heavens and the Earth 3. Cambridge University Press. 1959: 39. ISBN 9780521058018.
- ^ Boardman, Harry. Table For Use in Computing Arcs, Chords and Versines. Chicago Bridge and Iron Company. 1930: 32.
- ^ 國家鐵路局發佈《線路曲線矢距測量儀檢定規程》等3項鐵道行業計量規程規範. 中国政府网, 中央人民政府. 2023-08-29 [2023-11-09].
- ^ Nair, P. N. Bhaskaran. Track measurement systems—concepts and techniques. Rail International (International Railway Congress Association, International Union of Railways). 1972, 3 (3): 159–166. ISSN 0020-8442. OCLC 751627806.
- Boyer, Carl B. A History of Mathematics 2nd. New York: Wiley. 1991.
- sagitta. 牛津英語詞典 (第三版). 牛津大學出版社. 2005-09 (英语).
- Miller, J. Earliest known uses of some of the words of mathematics (v). [2010-04-02]. (原始内容存档于2015-09-05).
- Calvert, James B. Trigonometry. [2010-04-02]. (原始内容存档于2007-10-02).
- haversine. 牛津英語詞典 (第三版). 牛津大學出版社. 2005-09 (英语).
- Cites coinage by Prof. Jas. Inman, D. D., in his Navigation and Nautical Astronomy, 3rd ed. (1835).
- Nair, Bhaskaran. Track measurement systems—concepts and techniques. Rail International. 1972, 3 (3): 159–166. ISSN 0020-8442.
- 埃里克·韦斯坦因. Haversine. MathWorld.























































![{\displaystyle {\begin{aligned}{\frac {\operatorname {versin} (\theta )+\operatorname {coversin} (\theta )}{\operatorname {versin} (\theta )-\operatorname {coversin} (\theta )}}-{\frac {\operatorname {exsec} (\theta )+\operatorname {excsc} (\theta )}{\operatorname {exsec} (\theta )-\operatorname {excsc} (\theta )}}&={\frac {2\operatorname {versin} (\theta )\operatorname {coversin} (\theta )}{\operatorname {versin} (\theta )-\operatorname {coversin} (\theta )}}\\[3pt][\operatorname {versin} (\theta )+\operatorname {exsec} (\theta )]\,[\operatorname {coversin} (\theta )+\operatorname {excsc} (\theta )]&=\sin(\theta )\cos(\theta )\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2309ac826855590e363f4f4e8372c556ac165e3e)



